A circle closes

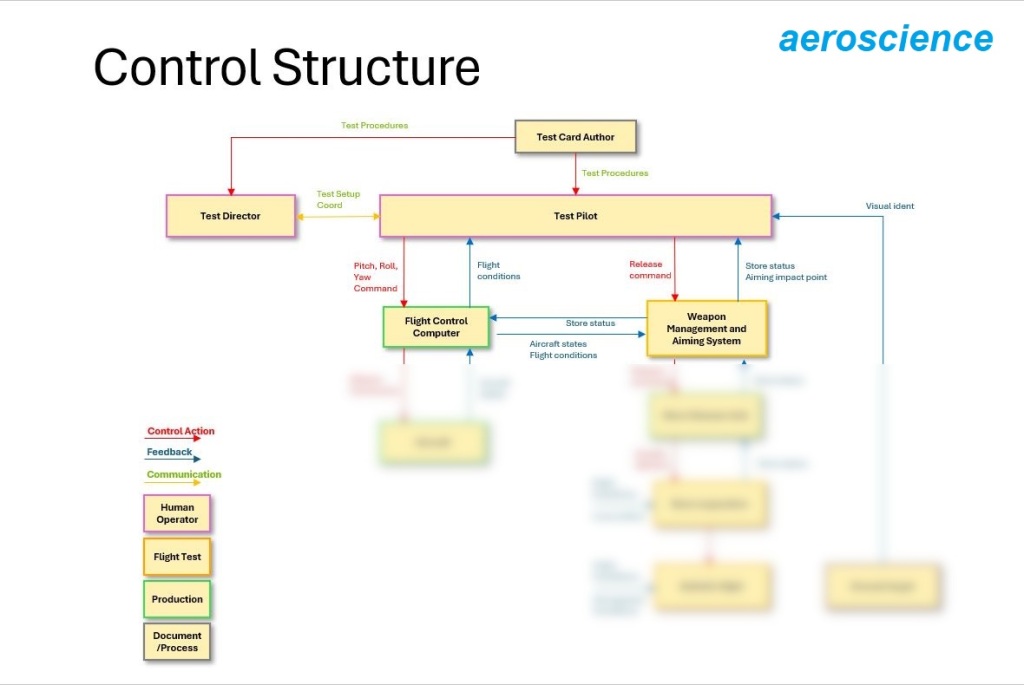
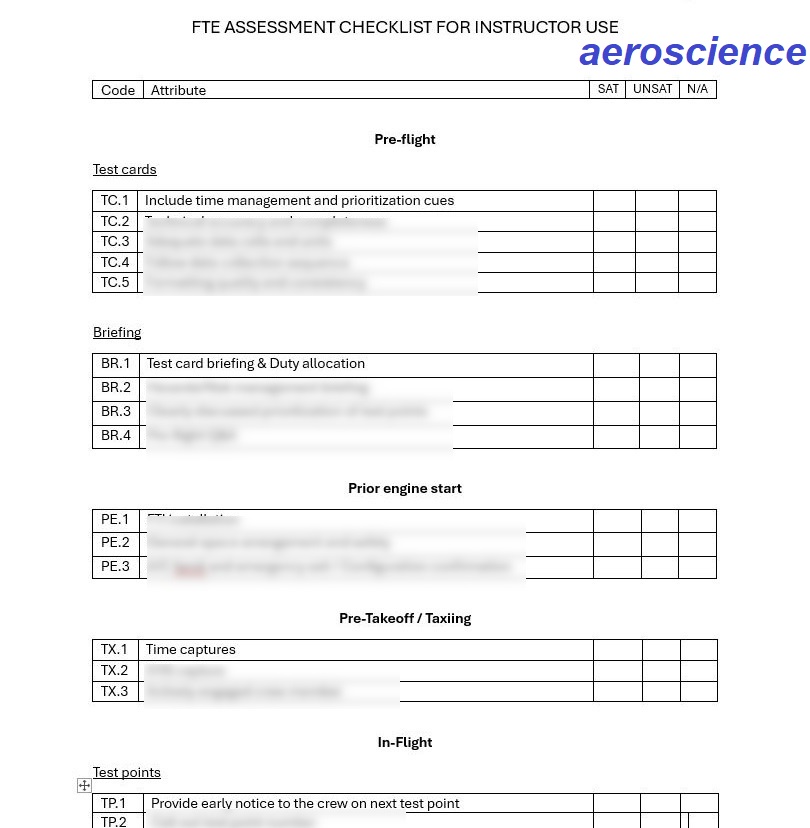
12 years ago when I decided to leave everything and join ITPS, there were some people who laughed. They said back then: “ITPS is not SETP recognized, it owns no aircraft, it has no permanent staff, it has no facilities, it has hardly run any long courses”. But I did not listen. I trusted Giorgio Clementi, seeing in him the passion for flight test and just followed my dream, not looking for job security or money or career achievements, but just looking to have fun!

ITPS was never a “9 to 5” job for me. It was just a fun thing to do, because it is always about having fun. It’s about looking forward to the next day of exciting things to do, new things to learn and new ways to challenge yourself.

It is because of fun that I am moving on to my next career step.
In the words of Charles Bukowski “There is no other way, and there never was…”

Canadian SFTE Chapter social

A very nice evening took place for the Canadian SFTE Chapter at the ITPS Sim center, where Members with their families enjoyed some social time and flying in the center’s flight simulators.

Trying the ITPS eVTOL sim. The FCS principles are there, some small improvements still required.

A short presentation on Chapter status and activities by the Chapter President and event organizer (me :)) was followed by pizza and a Flight Test documentary in the mission theater. Glad that everyone enjoyed!

Last week selfies

This has been my last full week at ITPS. I am posting a couple of selfies because I believe that they say something…

Last week after almost 12 years, and there are days that I am still the last one to leave the office…

My last flight. Flying as the examiner on the CFTE-I check-ride.

Introduction to STPA for Flight Test Safety

Last week I introduced the STPA method to ITPS curriculum, making ITPS one of the first TPSs, if not the first one, to teach this flight test safety method. STPA is particularly strong in novel and complex systems (like smart systems including autonomy and/or AI), as it provides the tester with a structured approach to identity causal scenarios leading to hazards and implement appropriate mitigation methods.

During the lectures we worked on two practical flight test cases with students, an Auto Takeoff, and a classic Store Separation. I’d like to thank Dunes, Darren and Jordan of Boeing Fight Test for reviewing the material and providing valuable feedback.

Coming soon…

Unbelievably excited! “Fingers crossed”…

FTE Assessment Checklist for Instructor use

As I have been assigned check rides of both student FTEs and Instructor FTEs (FTE-I) I felt something capturing the FTE attributes of the assessment was missing.

Created a checklist for that purpose and hold a meeting with FTE-I’s to refine. I think we came up with a useful tool.

Reviewer for AIAA Aviation 2024

For one more year I reviewed abstracts for the AIAA Aviation conference. Interesting to see publishable scientific results before everyone else. Keeps you on your toes…

(It’s been a while since my last blog post. Too busy with too many things…)

EASA LFTE certificate received

While I have been an instructor and examiner for the EASA LFTE course, I did not technically hold the certificate till now.

This can serve me in various ways going forward and provides formal proof of having received flight test training compliant with EASA. Part 21. Annex I. Appendix XII. AMC 1 (Compliance Level 1 – Aeroplane) – even if I think that it really relates to a very basic level compared to my 10+ years of flight test engineering experience at ITPS and 3 years as CAO.
I should also note that I am the only Greek who holds this certification (but I am not the only Canadian, see I recently also got my Canadian citizenship!)

STEM talk to 6th grade students

Inspiring younger generations towards science and engineering is not only a pleasure; it’s our duty.
I enjoyed so much talking to the 6th grade students of a Canadian Public school on aviation and space as part of a STEM event.
Truth is that grabbing (and maintaining) children’s attention is more challenging than it sounds, though being expressive and demonstrative helps a lot, especially if you are talking on airplanes and rockets!

WISA Summit 2023 & Bjarni

I attended the WISA Summit 2023 and Commander’s Chris Hadfield keynote talk was the best way to close the event. Hadfield ‘s talk was targeted around Sustainability in aviation and there was a slide on Bjarni’s flight on the Silver Dart.
Bjarni, I am sure you are smiling up there…